ANALYSIS

As described in Instrumentation and in Stone et. al. (1977) each of the Voyager space craft has four low-energy telescopes (LETs); two double-ended high-energy telescopes (HETs); and an electron telescope (TET). The telescopes are oriented at different viewing angles to provide information on energetic particle streaming patterns.

The LET and HET A stopping analyses generally use the dE/dx-E technique outlined for LET in Cook (1981) and Cook et.al. (1984) to measure the kinetic energy and the nuclear charge Z of individual incident nuclei. Each HET and LET are composed of a stack of cylindrical, solid-state detectors, the first two of which are thinner than the subsequent detectors and spaced apart from each other in order to establish a field of view.

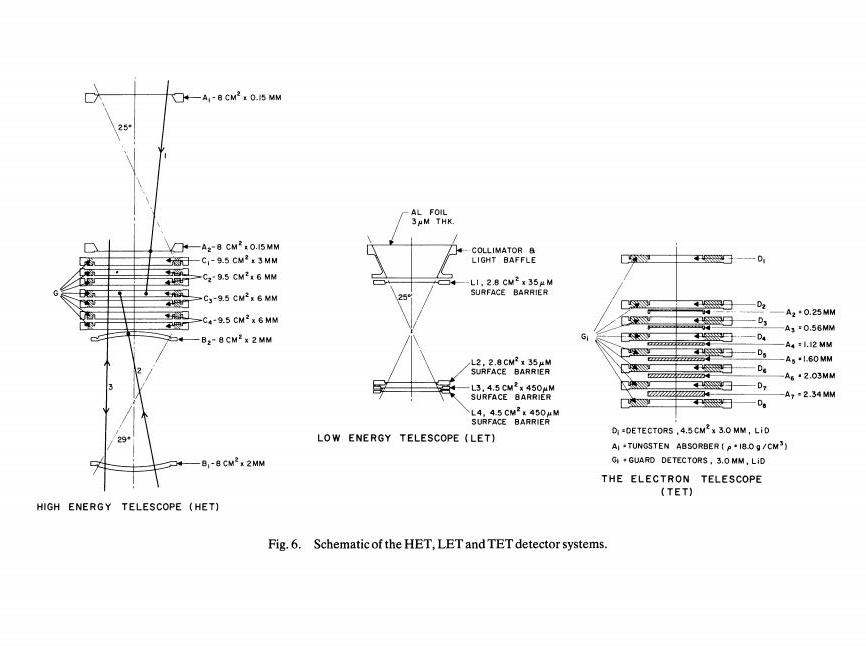
Three energy losses are recorded for each incident particle, one each from the first two detectors and the third represents the sum of the energy lost in the remaining detectors (one in the case of LET), except for the last detector, which is used in anti-coincidence to identify and eliminate penetrating particles. These three energy losses allow for two semi-independent determinations of the nuclear Z of the particle to be determined, provided the particle penetrates the first two detectors.

A consistency criterion is applied to these two determinations of Z to eliminate background events, and the average of the two Z determinations gives the estimated Z of the particle, which is generally not an integer. Using a model of the telescope, including the thickness of any window and/or thermal blanket material covering the entrance aperture and any inactive thickness of the detectors, incident energy per nucleon bins are mapped to energy loss bins in the active thicknesses of the detectors.

HET penetating H and He consists of particles that trigger detectors B1, B2, and C1. Three pulse heights are returned for each event in the form of channel numbers, which are approximately linearly related to the energy losses in the detectors. These pulse heights are from B1, C1, and C4+C3+C2 (C432). For each C432 channel applicable to the species (proton or helium nucleus), a response table contains limits of B1 channels, limits of C1 channels, and the highest incident energy that triggers this C432 channel. If the B1 and C1 pulse heights of an event fall within the B1 and C1 limits, respectively, for the C432 channel of a certain element, then this event is identified as belonging to that element, and its incident energy lies between the incident energy corresponding to the next lower C432 channel and that corresponding to C432 of the event.

For the response tables the applicable range of incident energies, energy losses along a mean trajectory through the telescope are computed using a range-energy relation. From these energy losses, mean energy losses in B1 and C1 for C432 energy losses corresponding to the C432 channel boundaries are located. Representative samples of flight data are examined to estimate the spread of B1 and C1 pulse heights about the mean values. These estimates are used to compute the limits on B1 and C1 for each C432 channel for each element.

The effect of nuclear interactions is accounted for in an approximate way. First, for protons it is assumed that the effect of interactions is negligible. For He, it is assumed there is an 11% reduction in He intensities due to nuclear interactions and that correction is accounted for by using different geometry factors for H as compared to He.